Temporal dynamics
dE/dt = F(E, n) = [A - (n/nb)M][1 - tanh(E - 3)]E2/2 - E,dn/dt = H(E, n) = ε [Θ(E - 1) - n].
where A = 1.5415.

Temporal dynamicsdE/dt = F(E, n) = [A - (n/nb)M][1 - tanh(E - 3)]E2/2 - E,dn/dt = H(E, n) = ε [Θ(E - 1) - n]. where A = 1.5415. |

|
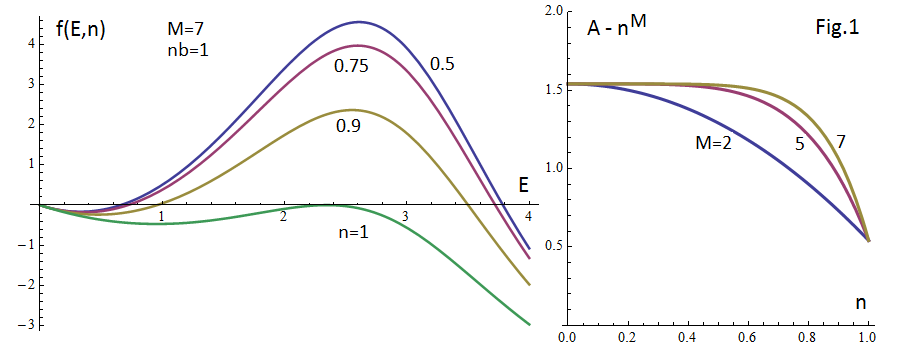
Below you can explore the model for different parameter values. To the left the phase plane of the system is shown. F(E,n) = 0 null cline is the red line and H(E,n) = 0 null cline is the green line. To the right E(t)/4 excitation (black) and n(t) recovery (blue) variables are plotted. The script makes 800 time steps dt.
M
nb
ε
dt
Eo
no
Ymax
field
The system makes an excitation cycle and goes to the stable fixed point
(E=0, n=0).
[1] Alain Karma
Spiral Breakup in Model Equations of Action Potential Propagation in
Cardiac Tissue
Phys.Rev.Lett. 71, 1103 (1993)