Bifurcation diagram for quadratic maps
There is a good way to trace bifurcations on the (x, c).
Let us plot iterations fc: xo = 0 →
x1 → x2 →...→ xMaxIt
for all real c. Colors (from blue to red)
show how often an orbit visits the pixel.
You can watch iterations of fc(x) for corresponding
c values on the right.
Controls: Click mouse to zoom in 2 times. Click mouse with
Ctrl to zoom out. Hold Shift key to zoom in the c
(vertical) direction only. Max number of iterations = 2000.
See coordinates of the image center and Δx, Δc in the
text field. The vertical line goes through x = 0.
The top part of the picture corresponds to a single attracting fixed point
of f for -3/4 < c < 1/4. For c > 1/4 points go away
to +∞ (see tangent bifurcation).
Filaments and broadening show how the critical orbit points are attracted to
the fixed point. Near c = -3/4 we see a branching point due to
period doubling bifurcation. Then all the
Feigenbaum's bifurcation cascade.
At the lower part of the bifurcation diagram you see chaotic bands and
white narrow holes of windows of periodic dynamics. The lowest and
biggest one corresponds to the period-3 window.
Compare the map with the Mandelbrot set to the right.
The bifurcation map patterns
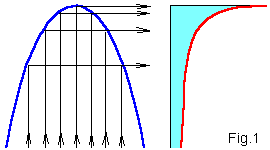 Fig.1 shows that caustics in distribution of points of chaotic orbits are
generated by an extremum of a mapping. Therefore singularities (painted in the
red) on the bifurcation diagram appear at images of the critical point
fc on(0).
Let us denote gn(c) = fcon(0), then
Fig.1 shows that caustics in distribution of points of chaotic orbits are
generated by an extremum of a mapping. Therefore singularities (painted in the
red) on the bifurcation diagram appear at images of the critical point
fc on(0).
Let us denote gn(c) = fcon(0), then
go(c) = 0, g1(c) = c,
g2(c) = c2 + c, ...
The curves g0,1,...,6(c) are shown in Fig.2.
Contents
Previous: Iterations of real maps
Next: Iterations on complex plane.
The M and J sets
updated 8 Sep 2013

 Fig.1 shows that caustics in distribution of points of chaotic orbits are
generated by an extremum of a mapping. Therefore singularities (painted in the
red) on the bifurcation diagram appear at images of the critical point
fc on(0).
Let us denote gn(c) = fcon(0), then
Fig.1 shows that caustics in distribution of points of chaotic orbits are
generated by an extremum of a mapping. Therefore singularities (painted in the
red) on the bifurcation diagram appear at images of the critical point
fc on(0).
Let us denote gn(c) = fcon(0), then
